elementui+Vue+OSS使用图片上传和删除
本文共 4294 字,大约阅读时间需要 14 分钟。
效果图1:
 效果图2:新增页面
效果图2:新增页面  效果图3:修改页面
效果图3:修改页面  首先引入依赖:
首先引入依赖: com.aliyun.oss aliyun-sdk-oss 3.8.0
在后台定义一个控制器:
package com.ff.controller;import com.aliyun.oss.OSSClient;import org.springframework.web.bind.annotation.CrossOrigin;import org.springframework.web.bind.annotation.RequestMapping;import org.springframework.web.bind.annotation.RestController;import org.springframework.web.multipart.MultipartFile;import java.io.IOException;import java.io.InputStream;import java.util.HashMap;import java.util.Map;import java.util.UUID;@RestController@RequestMapping("/file")@CrossOriginpublic class FileController { // 阿里云oss上传文件相关属性值 // 阿里云API的外网域名 public static final String ENDPOINT = "oss-cn-beijing.aliyuncs.com"; // 阿里云API的密钥 public static final String ACCESS_KEY_ID = "LTAI4Fzm3c6EmUexCHLvZNcm"; // 阿里云API的密钥Access Key Secret public static final String ACCESS_KEY_SECRET = "b8OrjTpYWW3sZSlimy4sxr2WJuNX09"; // 阿里云API的bucket名称 public static final String BACKET_NAME = "jjjqqq"; // 阿里云API的文件夹名称 public static final String FOLDER = "photo/"; public static final String URl = "https://jjjqqq.oss-cn-beijing.aliyuncs.com/"; //图片上传 @RequestMapping("uploadPhoto") public Map uploadPhoto(MultipartFile file) { Map map=new HashMap<>(); OSSClient ossClient = null; InputStream inputStream = null; try { ossClient = new OSSClient(ENDPOINT,ACCESS_KEY_ID,ACCESS_KEY_SECRET); //获取文件名 String filename = file.getOriginalFilename(); //截取后缀 String suffx = filename.substring(filename.lastIndexOf(".")); //获取文件名称 String newFileName= UUID.randomUUID()+suffx; inputStream = file.getInputStream(); ossClient.putObject(BACKET_NAME,FOLDER+newFileName,inputStream); map.put("filePath",URl+FOLDER+newFileName); } catch (Exception e) { e.printStackTrace(); }finally { if(inputStream!=null){ try { inputStream.close(); } catch (IOException e) { e.printStackTrace(); } } if(ossClient!=null){ ossClient.shutdown(); } } return map; }} App.Vue页面:
新增 批量删除 { { scope.$index+1}} ![]()
删除 编辑 ![]()
新增 修改 取消
其中img是自己定义的,数据库没有这个字段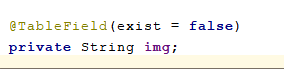
@Override @Override public void updateDrug(Drug drug) { //判断新图片和老图片都不为null if(StringUtils.isNotBlank(drug.getImg()) && StringUtils.isNotBlank(drug.getPhoto()) && !drug.getImg().equals(drug.getPhoto())){ //删除老图片 OSSUtil.deleteFile(drug.getImg()); } drugMapper.updateById(drug); } 其中OSSUtil是自己写的工具类:
public class OSSUtil { // 阿里云oss上传文件相关属性值 // 阿里云API的外网域名 public static final String ENDPOINT = "oss-cn-beijing.aliyuncs.com"; // 阿里云API的密钥 public static final String ACCESS_KEY_ID = "LTAI4Fzm3c6EmUexCHLvZNcm"; // 阿里云API的密钥Access Key Secret public static final String ACCESS_KEY_SECRET = "b8OrjTpYWW3sZSlimy4sxr2WJuNX09"; // 阿里云API的bucket名称 public static final String BACKET_NAME = "jjjqqq"; // 阿里云API的文件夹名称 public static final String FOLDER = "photo/"; public static final String URl = "https://jjjqqq.oss-cn-beijing.aliyuncs.com/"; public static void deleteFile(String fileName){ OSSClient ossClient = new OSSClient(ENDPOINT,ACCESS_KEY_ID,ACCESS_KEY_SECRET); //给fileName重新赋值 String replace = fileName.replace(URl, ""); //删除 ossClient.deleteObject(BACKET_NAME,replace); ossClient.shutdown(); }} 转载地址:http://jpljz.baihongyu.com/
你可能感兴趣的文章
MySQL相关命令
查看>>
mysql社工库搭建教程_社工库的搭建思路与代码实现
查看>>
Warning: Can't perform a React state update on an unmounted component. This is a no-
查看>>
mysql笔记 (早前的,很乱)
查看>>
MySQL笔记:InnoDB的锁机制
查看>>
mysql第一天~mysql基础【主要是DDL、DML、DQL语句,以及重点掌握存存引擎、查询(模糊查询)】
查看>>
mysql第二天~mysql基础【查询排序、分页查询、多表查询、数据备份与恢复等】
查看>>
MySQL简介和安装
查看>>
MySQL简单查询
查看>>
MySQL管理利器 MySQL Utilities 安装
查看>>
MySQL篇(管理工具)
查看>>
mysql类型转换函数convert与cast的用法
查看>>
mysql系列一
查看>>
MySQL系列之数据授权(安全)
查看>>
MySQL系列之数据类型(Date&Time)
查看>>
MySQL系列之数据类型(Date&Time)
查看>>
Mysql系列之锁机制
查看>>
Mysql系列九:使用zookeeper管理远程Mycat配置文件、Mycat监控、Mycat数据迁移(扩容)...
查看>>
MySql系列:[4200][1140]In aggregated query without GROUP BY, expression #2 of SELECT list contains nona
查看>>
MySQL索引
查看>>